Biological Electricity
Electrical
events in biological tissues are caused by the movement of ions across the membrane. A potential
difference exists across the membranes of all cells (membrane potential,
Em), but only excitable tissues can generate action
potentials (transient depolarization of a cell as a result of ion channel
activity). Action potentials transmit information in nerve cells (Chapter 6)
and trigger contractions in muscle cells (Chapter 12). Cell membranes are
electrically polarized so that the inside is negative relative to the outside.
In excitable tissues, resting Em is
usually between –60 and –90 mV.
The Resting Membrane Potential
The resting membrane is more
permeable to K+ and Cl– than to other ions (Chapter 4).
The cell contains negatively charged molecules (e.g. proteins) which cannot
cross the membrane. This fixed negative charge attracts K+, leading
to accumulation of K+ within the cell (Chapter 2). However, the
consequent increase in the K+ concentration gradient drives K+ back
out of the cell. This means fewer K+ ions move into the cell than are required
to achieve electrical neutrality with the fixed negative charges, and the
inside of the cell therefore remains negatively charged compared to the
outside, causing a potential difference across the membrane. Equilibrium is
reached when the electrical forces exactly balance those due to concentration
differences (Gibbs–Donnan equilibrium); the net force or electrochemical
gradient for K+ is then zero. If the membrane were only permeable to
K+, the voltage at which this would occur (K+ equilibrium potential,
EK) is defined purely by
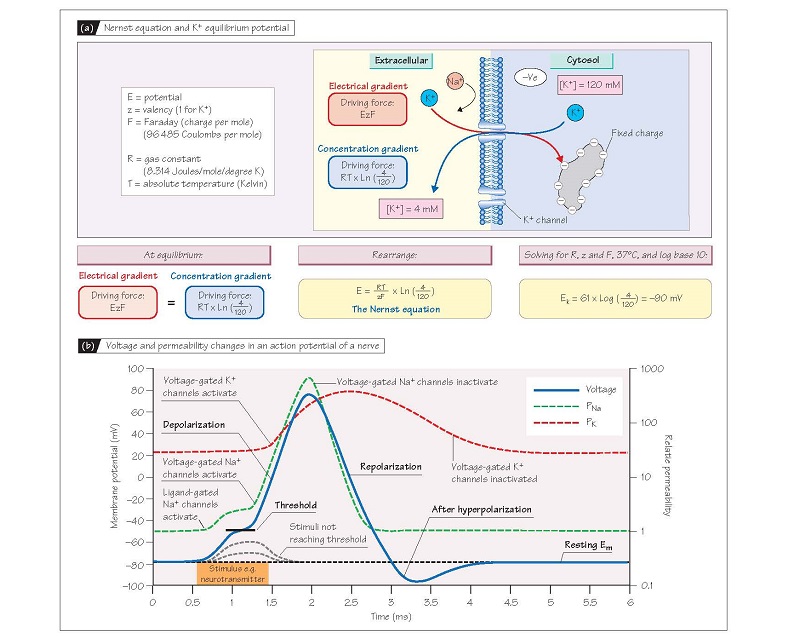
the K+ concentration gradient, and can be calculated from the Nernst
equation (see Fig. 5a for derivation). Thus, if intracellular [K+]
were 120 mmol/L and extracellular [K+] 4 mmol/L, EK = ∼–90 mV.
This applies to any ion, so if the membrane were only permeable to Na+
(only Na+ channels open) and intracellular and extracellular [Na+] were 10 and
140 mmol/L, respectively, the potential obtained at equilibrium (ENa)
would be +70 mV. To summarize, for any given intracellular and extracellular
ionic concentrations, the equilibrium potential for that ion is the membrane
potential required for the intracellular and extracellular concentrations to be
in equilibrium, i.e. for the electrochemical gradient to be zero. The
difference between the actual Em and the equilibrium potential for any
ion is therefore a measure of that ion’s electrochemical gradient, the force
driving it into or out of the cell.
Real cell membranes are permeable
to other ions besides K+, but at rest their K+ permeability (PK) is much
greater than that for other ions. In particular, the ratio of PK to Na+
permeability (PNa) ranges between 25 : 1 and 100 : 1 in nerve, skeletal
and cardiac muscle cells. As a result Em in such cells at rest (resting
membrane potential) is close to EK (–60 to –85 mV) and the
electrochemical gradient for K+ is small. Em does not equal EK
because there is permeability to other ions, notably Na+. As ENa is much
more positive than Em, the Na+ electro-chemical gradient
is strongly inwards,
forcing Na+ into
the cell.
However, as PNa is
relatively low, only a small amount of Na+ can leak in, though this is sufficient to slightly
depolarize the membrane from EK. A consequence of the above is that if PNa
were suddenly increased to more than PK, then Em would shift
towards ENa. This is exactly what happens during an action potential,
when Na+ channels open so that PNa becomes 10-fold greater than PK,
and the membrane depolarizes.
The action potential
Action potentials are initiated in
nerve and skeletal muscle by activation of ligand-gated Na+ channels by
neurotransmitters (Chapter 4 and 13). This increases PNa and causes Em
to move towards ENa (i.e. become positive; Fig. 5b). This initial
increase in PNa is however relatively modest, so the depolarization is
similarly small. However, if the stimulus is sufficiently strong, Em depolarizes enough to reach the threshold potential (∼-55 mV), at which point voltage-gated Na+
channels (Chapter 4) activate, causing further
depolarization. This activates
more voltage-gated Na+ channels so the process becomes explosively
self-regenerating, leading to a large transient increase in PNa so it is
10-fold greater than PK. As a result, Em rapidly approaches ENa
(∼+65 mV; see above), causing the sharp positive ‘spike’ or depo-
larization of the action potential, which lasts about 1 ms in nerve and
skeletal muscle. The spike is transient because as Em becomes positive,
the voltage-gated Na+ channels inactivate (Chapter 4) and PNa plummets,
whereas a type of voltage-gated K+ channel (delayed rectifier)
activates. Thus PK is again much larger than PNa and Em
returns towards EK (repolarization); this takes about 1–2 ms.
Delayed closure of the delayed rectifier K+ channels means that the PK:PNa
ratio remains transiently greater than normal after repolarization, causing a
transient hyperpolarization (Fig. 5b).
Following depolarization the Na+
channels remain inactive
for about 1 ms until the cell is largely repolarized and, during
this period, they cannot be opened by any amount of depolarization. This is
known as the absolute refractory period during which it is impossible to
generate another action potential. For the following 2–3 ms, the transient
hyperpolarization renders the cell more difficult to depolarize, an interval
known as the relative refractory period, when an action potential can be
generated only in response to a larger than normal stimulus. The refractory
period limits the frequency at which action potentials can be generated to
<1000/s and ensures that, once initiated, an action potential can travel
only in one direction. Once triggered, an action potential will travel over the
entire surface of an excitable cell (it is propagated) and will always have
the same amplitude (it is all- or-nothing). The minute changes in ion
concentrations that occur during an action potential are restored by the action
of the Na+ pump; it is important
to understand that the action potential is not due to changes in ionic
concentrations, but to changes in ionic permeability. Note that action
potentials in cardiac muscle differ somewhat from those in nerves and skeletal muscle (Chapter
19).